The Aalto University homepage of Teijo Arponen
My research interests are from quite a broad selection, both Pure and
Applied Mathematics.
Typically I classify myself as a numerical analyst with a specialty
in
numerical solution of ODEs (Ordinary Differential Equations). But my
true passion is combining different areas of mathematics.
Firstly, links related to my position at TKK (previously known as
"HUT"):
Secondly, links to people who I consider having been my mentors and can
give
further information about my work:
Thirdly, general links to some interesting research groups on
geometrical numerical integration (an incomplete list):
Research topics, latest first:
- (Singularities of) Multibody systems. This is continuation to my PhD topic (for
which see below). Collaborators in alphabetical order: Villesamuli
Normi, Samuli Piipponen, and Jukka Tuomela.
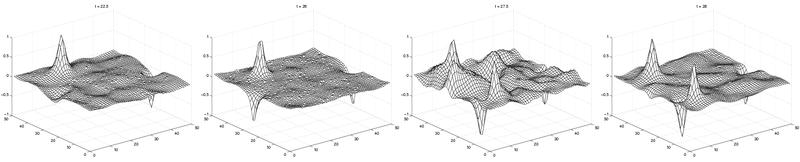
- Mathematical modelling using Hamiltonian systems with
thermostats. Collaboration with Ben Leimkuhler. As an application we
simulated ferromagnetic materials as spin lattices. This is a PDE
which is space-discretized to get a large ODE. The algorithm has also
been parallelized using OpenMP (with C). Some snapshots with periodic
boundary conditions:
- Using tensors in geometric numerical integration. The
principle behind geometric numerical integration is that some
geometrical structure (a priori known) is conserved (up to round-off
error) during the numerical discretization. This has been essential
for ability of integration over long time intervals. The most
successful approaches use either symplecticness or time-reversibility.
It is interesting to note, when one looks more closely, all of the
abovementioned structures are such that in addition to their
geometrical meaning there exists also an equivalent algebraic
representation of them. This is essential: we can find lots of
geometrical structures, but without an algebraic representation they
are of little use in algorithms. We need to work with geometry at the
background, algebra on the foreground! My tensor approach starts from
the other end: they are algebraic objects, not all of them are
important but those which have geometrical meaning.
Full text
article (via ScienceDirect).
- The topic of my PhD: Structural Analysis and Numerical
Solution of Differential-Algebraic Equations. The approach is based
on their involutive (or complete) form. Introduction and summary are
here.
(Unfortunately, copyright restrictions prevent me from giving the
whole Thesis.) Advisor
Jukka
Tuomela.
- Matrix approach to univariate polynomials.
Part I.
Part II.
- "Two-sided search" techniques in AI (artificial intelligence).
Collaboration with David Sarne, Harvard University, Boston USA. (No
preprints here, please send an email request if you are interested.)
Teaching (i.e. lecturing)
In the University of Warwick,
U.K., 2004-2005:
In the Aalto University (formerly known as Helsinki University of Technology):
- S1, Basic Course in
Mathematics 1 for MSc in Electrical Engineering
- K1, Basic Course in
Mathematics 1 for MSc in Civil Engineering
- P2, Basic Course in
Mathematics 2 for MSc in Chemical Engineering
- S2, Basic Course in
Mathematics 2 for MSc in Electrical Engineering
- V2,
Basic Course in Mathematics 2 for MSc in Engineering (multidisciplinary)
- KP3-II, Basic Course in
Mathematics 3 for MSc in Chemical Engineering
- L4, Basic Course in
Mathematics 4 for MSc with Extended Curriculum in Science
- Numerical and
Symbolic Computation (Maple and Matlab)
- Numerical
Linear Algebra (direct and iterative methods)
- Multibody Systems and
Numerics
- Finite Difference Methods,
- Numerics
of Hamiltonian Systems
The topics of the "basic courses" include complex numbers, linear
algebra, univariate and multivariate calculus, numerical integration,
ordinary differential equations, analytic geometry in 2D and 3D,
series, curvilinear coordinates.
This page is maintained by Teijo.Arponen at aalto.fi.
Last updated April 12th, 2012.