Aalto Mathematics and Arts Colloquium
Prof. Daina Taimina (Cornell University): Study mathematics and ... become an artist?
Tuesday 16th May, 15:00-16:00
Aalto University, Otakaari 1, Hall C (Y205), Espoo
Chair: Martti Raevaara (Aalto)
Joint Mathematics and Arts Colloquium and Women in Mathematics Colloquium
When I was in middle school I was a good student and had very good grades in all subjects...except in art class where I was struggling to draw the way my teacher thought was the right way. I accepted my teacher's judgement that I can do anything but art and went to study mathematics. Could I imagine that some fifty years later I will be known as an artist? Mathematics and art are not too distant. In my talk, I will discuss the interplay between mathematics and art from my own perspective.
More information on Professor Taimina's art and research: see her homepage and blog.
Past talks:
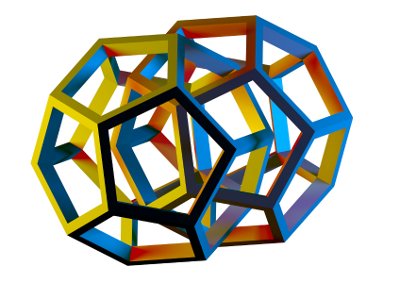
Henry Segerman: Design of 3D printed mathematical art
Wednesday 15th March, 16:00-17:00
Aalto University, Otakaari 1, Hall B, Espoo
Chair: Anna Valtonen (Aalto)
When visualising topological objects via 3D printing, we need a
three-dimensional geometric representation of the object. There are
approximately three broad strategies for doing this: "Manual" - using
whatever design software is available to build the object by hand;
"Parametric/Implicit" - generating the desired geometry using a
parametrisation or implicit description of the object; and "Iterative"
- numerically solving an optimisation problem.
The manual strategy is unlikely to produce good results unless the
subject is very simple. In general, if there is a reasonably canonical
geometric structure on the topological object, then we hope to be able
to produce a parametrisation of it. However, in many cases this seems
to be impossible and some form of iterative method is the best we can
do. I will discuss these matters with many examples, including
visualisation of four-dimensional polytopes (using orthogonal versus
stereographic projection) and Seifert surfaces (comparing my work with
Saul Schleimer with Jack van Wijk's iterative techniques).
I will also describe some computational problems that have come up in
my 3D printed work, including the design of 3D printed mobiles (joint
work with Marco Mahler), "Triple gear" and a visualisation of the
Klein Quartic (joint work with Saul Schleimer), and hinged surfaces
with negative curvature (joint work with Geoffrey Irving).
Henry Segerman: 3D Shadows: Casting light on the fourth dimension
Thursday 16th March, 18:30-19:30
Tiedekeskus Heureka, Auditorio, Kuninkaalantie 7, Vantaa
Chair: Jari Jokinen (TEK)
Our brains have evolved in a three-dimensional environment, and so we are very good at visualising two- and three-dimensional objects. But what about four-dimensional objects? The best we can really do is to look at three-dimensional "shadows". Just as a shadow of a three-dimensional object squishes it into the two-dimensional plane, we can squish a four-dimensional shape into three-dimensional space, where we can then make a sculpture of it. If the four-dimensional object isn't too complicated and we choose a good way to squish it, then we can get a very good sense of what it is like. We will explore the sphere in four-dimensional space, the four-dimensional polytopes (which are the four-dimensional versions of the three-dimensional polyhedra), and various 3D printed sculptures, puzzles, and virtual reality experiences that have come from thinking about these things. I talk about these topics and much more in my new book, "Visualizing Mathematics with 3D Printing".
See the video recording of the talk at Aalto Youtube channel.
No registration, everyone is welcome!
For additional information, please contact Kirsi Peltonen.
